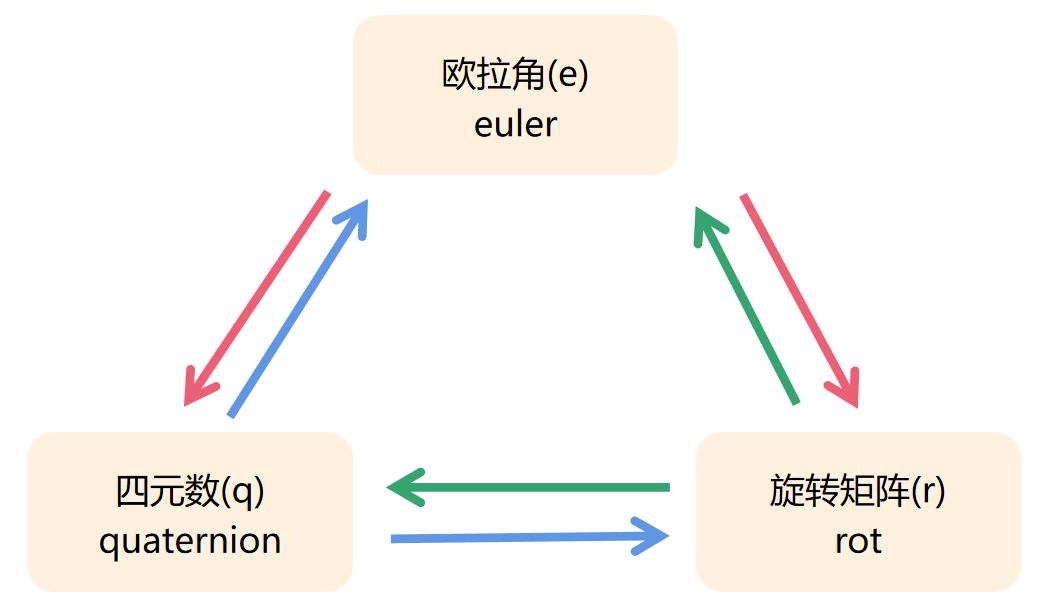
欧拉角,四元数和旋转矩阵互转代码【python版】
代码包括了`欧拉角与四元数互转`,`旋转矩阵与四元数互转`,`欧拉角与旋转矩阵互转`,输入参数均为`np.array`形式代码内置了角度制和弧度制😃😃,当时因为这块吃了好多亏里面有验证函数
写在前面
- 欧拉角以Roll、Pitch、Yaw的顺序表示
- 四元数以[ q w q_w qw, q x q_x qx, q y q_y qy, q z q_z qz]的顺序表示
- 代码包括了
欧拉角与四元数互转,旋转矩阵与四元数互转,欧拉角与旋转矩阵互转,输入参数均为np.array形式 - 代码内置了角度制和弧度制😃😃
当时因为这块吃了好多亏 - 顺便测试了一下pydrake库,发现:
- pydrake库中是弧度制
- 输出结果与代码输出结果几乎一致(但pydrake精度更高)
- 由于原理这块肯定已经有很多很完善的资料了所以不做过多赘述
思路
东拼西凑找出的这些公式,为了验证他们的正确性,采用以下方法验证:
随机生成一个欧拉角e,将其与
a. 欧拉角e -> 旋转矩阵r -> 欧拉角e;
b. 欧拉角e -> 四元数q -> 欧拉角e;
c. 欧拉角e -> 四元数q -> 旋转矩阵r -> 欧拉角e;
d. 欧拉角e -> 旋转矩阵r -> 四元数q -> 欧拉角e
比较,看误差有多大(如图所示),并重复n轮

直接上代码!
注意⚠️
涉及到旋转矩阵的四个函数似乎有点问题【虽然自洽但是结果不对!】
如果最后导出的是旋转矩阵,请暂时不要使用本代码!!!
红豆泥私密马赛!
代码部分
utils.py
import math
import numpy as np
# from pydrake.all import RotationMatrix, RollPitchYaw
# 四元数转欧拉角
# ================OKOK
def quaternion_to_euler(q, degree_mode=1):
qw, qx, qy, qz = q
roll = math.atan2(2 * (qw * qx + qy * qz), 1 - 2 * (qx ** 2 + qy ** 2))
pitch = math.asin(2 * (qw * qy - qz * qx))
yaw = math.atan2(2 * (qw * qz + qx * qy), 1 - 2 * (qy ** 2 + qz ** 2))
# degree_mode=1:【输出】是角度制,否则弧度制
if degree_mode == 1:
roll = np.rad2deg(roll)
pitch = np.rad2deg(pitch)
yaw = np.rad2deg(yaw)
euler = np.array([roll, pitch, yaw])
return euler
# 欧拉角转四元数
# ================OKOK
def euler_to_quaternion(euler, degree_mode=1):
roll, pitch, yaw = euler
# degree_mode=1:【输入】是角度制,否则弧度制
if degree_mode == 1:
roll = np.deg2rad(roll)
pitch = np.deg2rad(pitch)
yaw = np.deg2rad(yaw)
qx = np.sin(roll/2) * np.cos(pitch/2) * np.cos(yaw/2) - np.cos(roll/2) * np.sin(pitch/2) * np.sin(yaw/2)
qy = np.cos(roll/2) * np.sin(pitch/2) * np.cos(yaw/2) + np.sin(roll/2) * np.cos(pitch/2) * np.sin(yaw/2)
qz = np.cos(roll/2) * np.cos(pitch/2) * np.sin(yaw/2) - np.sin(roll/2) * np.sin(pitch/2) * np.cos(yaw/2)
qw = np.cos(roll/2) * np.cos(pitch/2) * np.cos(yaw/2) + np.sin(roll/2) * np.sin(pitch/2) * np.sin(yaw/2)
q = np.array([qw, qx, qy, qz])
return q
# 四元数转旋转矩阵:
def quaternion_to_rot(q):
q0,q1,q2,q3=q
R=np.array([[1-2*(q2**2+q3**2),2*(q1*q2-q3*q0),2*(q1*q3+q2*q0)],
[2*(q1*q2+q3*q0),1-2*(q1**2+q3**2),2*(q2*q3-q1*q0)],
[2*(q1*q3-q2*q0),2*(q2*q3+q1*q0),1-2*(q1**2+q2**2)]])
return R
# 旋转矩阵转四元数:
# ================OKOK
def rot_to_quaternion(R):
R=R
qw=np.sqrt(1+R[0,0]+R[1,1]+R[2,2])/2
qx=(R[2,1]-R[1,2])/(4*qw)
qy=(R[0,2]-R[2,0])/(4*qw)
qz=(R[1,0]-R[0,1])/(4*qw)
q = np.array([qw, qx, qy, qz])
return q
# 旋转矩阵转欧拉角
# ================OKOK
def rot_to_euler(R, degree_mode=1):
sy = np.sqrt(R[0,0] * R[0,0] + R[1,0] * R[1,0])
singular = sy < 1e-6
if not singular :
roll = np.arctan2(R[2,1] , R[2,2])
pitch = np.arctan2(-R[2,0], sy)
yaw = np.arctan2(R[1,0], R[0,0])
else :
roll = np.arctan2(-R[1,2], R[1,1])
pitch = np.arctan2(-R[2,0], sy)
yaw = 0
# degree_mode=1:【输出】是角度制,否则弧度制
if degree_mode == 1:
roll = np.rad2deg(roll)
pitch = np.rad2deg(pitch)
yaw = np.rad2deg(yaw)
euler = np.array([roll, pitch, yaw])
return euler
# 欧拉角转旋转矩阵
# # ================OKOK
def euler_to_rot(euler, degree_mode=1):
roll, pitch, yaw = euler
# degree_mode=1:【输入】是角度制,否则弧度制
if degree_mode == 1:
roll = np.deg2rad(roll)
pitch = np.deg2rad(pitch)
yaw = np.deg2rad(yaw)
R_x = np.array([
[1, 0, 0 ],
[0, math.cos(roll), -math.sin(roll)],
[0, math.sin(roll), math.cos(roll) ]
])
R_y = np.array([
[math.cos(pitch), 0, math.sin(pitch) ],
[0, 1, 0 ],
[-math.sin(pitch), 0, math.cos(pitch) ]
])
R_z = np.array([
[math.cos(yaw), -math.sin(yaw), 0],
[math.sin(yaw), math.cos(yaw), 0],
[0, 0, 1]
])
R = np.dot(R_z, np.dot( R_y, R_x ))
return R
if __name__ == "__main__":
# 测试思路:随机生成几组欧拉角,用各种转换后看是否与原数据相同
# 备注:np.sin(),math.sin()的返回值都是弧度制
# np.atan2(), math.asin()这些也是弧度制
print(np.sin(90), np.sin(45))
print(np.sin(3.14), np.sin(1.57))
print(math.sin(90), math.sin(45))
print(math.sin(3.14), math.sin(1.57))
mode = 0 # 当这个值等于1时, 输入和输出的欧拉角均为 <角度制>
for i in range(10):
print('=' * 32, "index:", i, '=' * 35)
euler = np.random.randint(0, 360, 3) - 180
# euler = np.array([60, 30, 45])
if mode != 1:
# 限制最多小数点后三位
euler = np.array([round(t * math.pi / 180, 3) for t in euler])
print("euler = ", euler)
e2q2e = quaternion_to_euler(euler_to_quaternion(euler, mode), mode)
e2r2e = rot_to_euler(euler_to_rot(euler, mode), mode)
e2r2q2e = quaternion_to_euler(rot_to_quaternion(euler_to_rot(euler, mode)), mode)
e2q2r2e = rot_to_euler(quaternion_to_rot(euler_to_quaternion(euler, mode)), mode)
# 限制这些值为[-180,179)
if mode == 1:
e2q2e = [(int((180 * 3 + t)) % 360) - 180 for t in e2q2e]
e2r2e = [(int((180 * 3 + t)) % 360) - 180 for t in e2r2e]
e2r2q2e = [(int((180 * 3 + t)) % 360) - 180 for t in e2r2q2e]
e2q2r2e = [(int((180 * 3 + t)) % 360) - 180 for t in e2q2r2e]
else: # 限制最多小数点后三位
e2q2e = [round((int((math.pi * 3 * 1000 + t * 1000)) % 6283 - 3142) / 1000, 3)\
for t in e2q2e]
e2r2e = [round((int((math.pi * 3 * 1000 + t * 1000)) % 6283 - 3142) / 1000, 3)\
for t in e2r2e]
e2r2q2e = [round((int((math.pi * 3 * 1000 + t * 1000)) % 6283 - 3142) / 1000, 3)\
for t in e2r2q2e]
e2q2r2e = [round((int((math.pi * 3 * 1000 + t * 1000)) % 6283 - 3142) / 1000, 3)\
for t in e2q2r2e]
print("e -> q -> e = ", e2q2e, "norm of delta = ", np.linalg.norm(e2q2e - euler))
print("e -> r -> e = ", e2r2e, "norm of delta = ", np.linalg.norm(e2r2e - euler))
print("e -> r -> q -> e = ", e2r2q2e, "norm of delta = ", np.linalg.norm(e2r2q2e - euler))
print("e -> q -> r -> e = ", e2q2r2e, "norm of delta = ", np.linalg.norm(e2q2r2e - euler))
# if mode == 0:
# # pydrake中的旋转角是弧度制
# print("drake actual rot = ", RotationMatrix(RollPitchYaw(euler[0],euler[1],euler[2])))
# print("rot =", euler_to_rot(euler, mode))
# print("e -> q -> rot =", quaternion_to_rot(euler_to_quaternion(euler, mode)))
那串看起来非常笨重的限制我感觉我玷污了这个语言的美、、
一个问题
-
使用随机数,会发现部分随机的欧拉角会变成另一个角,这两个角的效果应该是等效的
吧

(使用弧度制也是一样) -
不过个人感觉在实际应用中应该也不会出现这种"错误的角度",因为角度都是通过各种正确值之间的转换得来的
补充
这里感谢下这个博主——
下面这几个网站都是从他的这篇文章转过来的👇
工具网站推荐 - 欧拉角四元数在线可视化转化网站/三维在线旋转变换网站
1 欧拉角四元数在线可视化转换网站
内容:四元数与欧拉角的可视化
特点:直观,明显
缺点:没有旋转矩阵
网站地址:https://quaternions.online/

2 三维在线旋转变换网站
内容:四元数与欧拉角与旋转矩阵的转换
特点:全面,丰富,可以调欧拉角形式
缺点:有一些并不常用的参数,容易搞混懵圈
网站地址:https://www.andre-gaschler.com/rotationconverter/

补充一点关于上面的问题
- 输入这个"错误欧拉角"在两个网站里也会得到一个不同的欧拉角,见上图和下图,所以应该不影响,也就是说正常使用时不会出现这种"错误欧拉角"


开放原子开发者工作坊旨在鼓励更多人参与开源活动,与志同道合的开发者们相互交流开发经验、分享开发心得、获取前沿技术趋势。工作坊有多种形式的开发者活动,如meetup、训练营等,主打技术交流,干货满满,真诚地邀请各位开发者共同参与!
更多推荐
 已为社区贡献6条内容
已为社区贡献6条内容





所有评论(0)