一阶RC低通滤波电路微分方程式推导

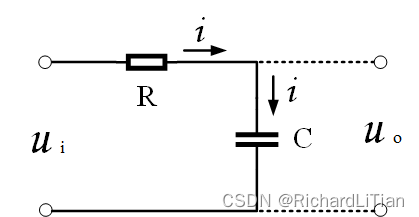
RC一阶低通滤波电路图如下图所示，

根据基尔霍夫电流定理，由图可知，
$$\begin{array}{r}i=C\frac{d{u}_0}{dt}\end{array}$$
根据基尔霍夫电压定理，由图可知，
$$\begin{array}{rl}& \\ RC\frac{d{u}_0}{dt}+u_0& =u_i\end{array}$$
将式子化为一阶线性微分方程，可得
$$\begin{array}{rl}& \\ \frac{d{u}_0}{dt}+\frac{1}{RC}{u}_0& =\frac{u_i}{RC}\end{array}$$

一阶线性方程微分的推导

接下来我们复习一下一阶线性微分方程通解的推导。
形如
$$\begin{array}{rl}\frac{dy}{dx}+P(x)y& =Q(x)\end{array}$$
的方程，称为一阶线性微分方程。
其中，$P(x)$、$Q(x)$均为$x$的已知函数，$Q(x)$称为已知项。
① 当$Q(x)=0$时，方程 $y{}^{\prime }+P(x)y=0$，此时方程为一阶齐次线性微分方程，那么
$$\begin{array}{rl}\frac{dy}{dx}+P(x)y& =0\\ \frac{dy}{y}& =-P(x)dx\end{array}$$
两边积分，可得
$$\begin{array}{rl}lny& =-{\int }_{}^{}P(x)dx+ln{C}_1\end{array}$$
求得通解为
$$\begin{array}{rl}y& =C_1{e}^{-{\int }_{}^{}P(x)dx}\end{array}$$
② 当 $Q(x)\ne 0$ 时，方程 $y{}^{\prime }+P(x)y=Q(x)$，此时方程为一阶非齐次线性微分方程，那么
$$\begin{array}{rl}\frac{dy}{dx}+P(x)y& =Q(x)\\ \frac{dy}{y}& =[-P(x)+\frac{Q(x)}{y}]dx\end{array}$$
两边积分，可得
$$\begin{array}{rl}lny& =-{\int }_{}^{}P(x)dx+{\int }_{}^{}\frac{Q(x)}{y}dx+ln{C}_2\end{array}$$
求得通解为
$$\begin{array}{rl}y& =C_2{e}^{{\int }_{}^{}\frac{Q(x)}{y}dx}\bullet e^{{\int }_{}^{}-P(x)dx}\end{array}$$
设 $C_2{e}^{{\int }_{}^{}\frac{Q(x)}{y}dx}=C(x)$
则有
$$\begin{array}{rl}y& =C(x)e^{{\int }_{}^{}-P(x)dx}\\ y{}^{\prime }& =C{}^{\prime }(x)e^{{\int }_{}^{}-P(x)dx}-C(x)P(x)e^{{\int }_{}^{}-P(x)dx}\end{array}$$
代入原方程 $y{}^{\prime }+P(x)y=Q(x)$ 中，得
$$\begin{array}{rl}C{}^{\prime }(x)e^{{\int }_{}^{}-P(x)dx}-C(x)P(x)e^{{\int }_{}^{}-P(x)dx}+P(x)C(x)e^{{\int }_{}^{}-P(x)dx}& =Q(x)\\ C{}^{\prime }(x)e^{{\int }_{}^{}-P(x)dx}& =Q(x)\\ C{}^{\prime }(x)& =Q(x)e^{{\int }_{}^{}P(x)dx}\end{array}$$
两边积分，得
$$\begin{array}{rl}C(x)& ={\int }_{}^{}Q(x)e^{{\int }_{}^{}P(x)}dx+C_3\end{array}$$
③ 综合一阶齐次线性微分方程 $y{}^{\prime }+P(x)y=0$ 的通解 $y=C_1{e}^{-{\int }_{}^{}P(x)dx}$
和一阶非齐次线性微分方程 $y{}^{\prime }+P(x)y=Q(x)$的通解 $y=C(x)e^{{\int }_{}^{}-P(x)dx}$，
可得，方程 $y{}^{\prime }+P(x)y=Q(x)$ 的通解公式为
$$\begin{array}{rl}y& =C_1{e}^{-{\int }_{}^{}P(x)dx}+C(x)e^{{\int }_{}^{}-P(x)dx}\\ y& =e^{-{\int }_{}^{}P(x)dx}[C(x)+C_1]\\ y& =e^{-{\int }_{}^{}P(x)dx}[{\int }_{}^{}Q(x)e^{{\int }_{}^{}P(x)dx}dx+C_3+C_1]\\ y& =(C_1+C_3)e^{-{\int }_{}^{}P(x)dx}+e^{-{\int }_{}^{}P(x)dx}{\int }_{}^{}Q(x)e^{{\int }_{}^{}P(x)dx}dx\end{array}$$

RC一阶低通滤波电路方程的推导

一阶线性微分方程的公式推导完毕，我们回到 RC一阶低通滤波电路方程
$$\begin{array}{rl}& \\ \frac{d{u}_0}{dt}+\frac{1}{RC}{u}_0& =\frac{u_i}{RC}\end{array}$$
由上文所推导的一阶线性微分方程的通解公式，可得
$$\begin{array}{rl}{u}_0& =C_0{e}^{-{\int }_{}^{}\frac{1}{RC}dt}+e^{-{\int }_{}^{}\frac{1}{RC}dt}({\int }_{}^{}\frac{u_i}{RC}{e}^{{\int }_{}^{}\frac{1}{RC}dt}dt)\\ & =C_0{e}^{-\frac{t}{RC}}+e^{-\frac{t}{RC}}({\int }_{}^{}\frac{u_i}{RC}{e}^{\frac{t}{RC}}dt)\\ & =C_0{e}^{-\frac{t}{RC}}+e^{-\frac{t}{RC}}({\int }_{}^{}{u}_{i}d{e}^{\frac{t}{RC}})\end{array}$$
到这一步，需要用到分部积分法，即 ${\int }_{}udv=uv-{\int }_{}vdu$
这里我们分析RC滤波电路，是想分析电源上电的那一刻，RC电路的特性，所以 $u_i$ 是阶跃信号，并且是单位阶跃函数，其表达式如下，
$$\begin{array}{rl}{u}_i(t)& =0,t＜0\\ u_i(t)& =1,t\ge 0\end{array}$$
其函数图象如下图所示，

阶跃信号 $u_i$ 的微分是冲激函数 ${\delta }_i$ ，如下图所示，

阶跃函数 $u_i$ 与冲激函数 ${\delta }_i$ 有如下关系和性质：
$$\begin{array}{rl}{\int }_{-\infty }^t{\delta }_i(\tau )d\tau & =u_i(t)\\ {\int }_{-\infty }^{+\infty }{\delta }_i(t)dt& =1\\ {\int }_{-0}^{+0}{\delta }_i(t)dt& =1\end{array}$$
所以，原函数可以继续往下推导如下
$$\begin{array}{rl}& \\ u_0& =C_0{e}^{-\frac{t}{RC}}+e^{-\frac{t}{RC}}({\int }_{}^{}{u}_{i}d{e}^{\frac{t}{RC}})\\ & =C_0{e}^{-\frac{t}{RC}}+e^{-\frac{t}{RC}}[u_i{e}^{\frac{t}{RC}}-{\int }_{}^{}{\delta }_i(t)e^{\frac{t}{RC}}dt]\end{array}$$
由阶跃信号的图象可知 $u_i$ 在 $t\ge 0$ 的时候恒为1，在 $t<0$ 时恒为0，因为 $RC$ 电路只在 $t\ge 0$ 时才有实际的物理意义，所以 $u_i{e}^{\frac{t}{RC}}=e^{\frac{t}{RC}}$ 。
对于冲激函数 ${\delta }_i$ 的积分，需要用到冲激函数的采样特性，如下：$$\begin{array}{rl}{\int }_{-\infty }^{+\infty }f(t)\delta (t)dt& =f(0)\\ {\int }_{-\infty }^{+\infty }f(t)\delta (t-t_0)dt& =f(t_0)\end{array}$$
所以 ${\int }_{}^{}{\delta }_i(t)e^{\frac{t}{RC}}dt=e^{\frac{0}{RC}}dt=1$
所以，原函数可以继续往下推导如下
$$\begin{array}{rl}& \\ u_0& =C_0{e}^{-\frac{t}{RC}}+e^{-\frac{t}{RC}}[u_i{e}^{\frac{t}{RC}}-{\int }_{}^{}{\delta }_i(t)e^{\frac{t}{RC}}dt]\\ & =C_0{e}^{-\frac{t}{RC}}+e^{-\frac{t}{RC}}(e^{\frac{t}{RC}}-e^{\frac{0}{RC}})\\ & =C_0{e}^{-\frac{t}{RC}}+1-e^{-\frac{t}{RC}}\\ & =(C_0-1)e^{-\frac{t}{RC}}+1\end{array}$$
推导到这一步，终于确定见到了 $u_0$ 与 $RC$ 的函数关系的庐山真面目，但此时还有一个常数 $C_0$ 需要确定。这时就需要把初状态的值 $t=0，u_0=0$ 代入方程中，可得
$$\begin{array}{rl}& \\ u_0& =(C_0-1)e^{-\frac{t}{RC}}+1\\ 0& =(C_0-1)e^{-\frac{0}{RC}}+1\\ 0& =C_0-1+1\\ C_0& =0\end{array}$$
所以，
$$\begin{array}{rl}& \\ u_0& =1-e^{-\frac{t}{RC}}\end{array}$$
这就是RC一阶低通电路的输出电压 $u_0$ 关于时间 $t$ 的函数关系式。

Matlab 画出RC一阶低通滤波器的函数曲线

得到了RC一阶低通电路的输出电压 $u_0$ 关于时间 $t$ 的函数关系式 $u_0=1-e^{-\frac{t}{RC}}$，对于式中的 $RC$ ，可以分别取值0.5，1，2，我们将这三个函数的曲线分别画出来，得到下图，



matlab代码如下：
t = 0:0.01:2; % 定义x轴范围
u = 1-exp(-t/0.5); % 定义y轴范围
plot(t,u); % 画图
xlabel(‘t’); % x轴标签
ylabel(‘u’); % y轴标签
title(‘一阶低通电路函数曲线（RC=0.5）’); % 图片标题
yticks(0:0.1:2) %y轴刻度

由 $u_0=1-e^{-\frac{t}{RC}}$ 的函数曲线可知，同样是在 $t=1.5$ 处取值，$RC=0.5$ 时，$u=0.95$ ；$RC=1$ 时，$u=0.77$ ；$RC=2$ 时，$u=0.52$ 。
由此可知，RC时间常数越小，则 $u_0$ 的上升时间就越短，也就是说 $u_0$ 能够更快地接近峰值 1。
RC时间常数的含义其实也很简单，就是当 $t=RC$ 时，$u_0=1-e^{-\frac{t}{RC}}=1-e^{-1}=1-\frac{1}{e}=1-0.3679=0.6321V$，$u_0$ 从 $0V$ 上升到 $0.6321V$ 或是从 $0V$ 上升到电源电压的 $63.21\%$ 所用的时间就是时间常数 $RC$ 的值，因为不同的电路 $RC$ 的值不同，所以会导致从 $0V$ 上升到电源电压的 $63.21\%$ 所用的时间也不尽相同。

Modelsim 仿真

Modelsim仿真电路图如下图所示，我们选用 $R=1k\Omega ，C=1\mu F$，则 $RC=1k\times 1\mu \bullet s=1ms$ 。

示波器波形图如下，

由波形图实测可得，$u_0$ 从 $1.25mV$ 上升到 $633.048mV$ 用时 $1.005ms$ ，符合时间常数 $RC$ 的计算值。
我们也可以用Matlab再画一条 $RC=1ms$ 的函数曲线，代码如下：
t = 0:0.0001:0.002; % 定义x轴范围
u = 1-exp(-t/0.001); % 定义y轴范围
plot(t,u); % 画图
xlabel(‘t’); % x轴标签
ylabel(‘u’); % y轴标签
title(‘一阶低通电路函数曲线（RC=1ms）’); % 图片标题
yticks(0:0.1:2)

曲线图如下，

由曲线图也可得到，当 $u_0=0.6321V$ 时， $t=0.001s=1ms$，符合计算值。

总结

至此，我们对 $RC$ 一阶低通滤波器电路完成了数学推导和电路仿真，这其中包含有一阶线性微分方程的求解、电路原理的运用以及仿真工具的使用。 $RC$ 电路的运用非常广泛，在电路中的作用也非常重要，在分析 $RC$ 电路时，从原理上分析更有助于我们理解电路，进而改善和提升电路的性能。